
WAVES
Introduction to Waves
The Concept of Wave
Explain the concept of a wave
A wave is a disturbance that travels through a medium from one location to another location.
Consider a slinky wave as an example of a wave. When the slinky is stretched from end to end and is held at rest, it assumes a natural position known as the equilibrium or rest position.
The coils of the slinky naturally assume this position, spaced equally far apart. To introduce a wave into the slinky, the first particle is displaced or moved from its equilibrium or rest position. The particle might be moved upwards or downwards, forwards or backwards; but once moved, it is returned to its original equilibrium or rest position.
The act of moving the first coil of the slinky in a given direction and then returning it to its equilibrium position creates a disturbance in the slinky. We can then observe this disturbance moving through the slinky from one end to the other. If the first coil of the slinky is given a single back-and-forth vibration, then we call the observed motion of the disturbance through the slinky a slinky pulse.
A pulse is a single disturbance moving through a medium from one location to another location.
However, if the first coil of the slinky is continuously and periodically vibrated in a back-and-forth manner, we would observe a repeating disturbance moving within the slinky that endures over some prolonged period of time. The repeating and periodic disturbance that moves through a medium from one location to another is referred to as a wave.
A medium is a substance or material that carries the wave.
You have perhaps heard of the phrase news media. The news media refers to the various institutions (newspaper offices, television stations, radio stations, etc.) within our society that carry the news from one location to another. The news moves through the media.
The wave medium is not the wave and it doesn't make the wave; it merely carries or transports the wave from its source to other locations.
In the case of our slinky wave, the medium through that the wave travels is the slinky coils. In the case of a water wave in the ocean, the medium through which the wave travels is the ocean water. In the case of a sound wave moving from the church choir to the pews, the medium through which the sound wave travels is the air in the room.
The Terms Wave Length, Frequency and Velocity of a Wave
Explain the terms wave length, frequency and velocity of a wave
Consider the transverse wave below:
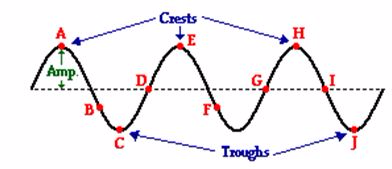
- A transverse wave is a wave in which the particles of the medium are displaced in a direction perpendicular to the direction of energy transport.
- The crest of a wave is the point on the medium that exhibits the maximum amount of positive or upward displacement from the rest position.
- The trough of a wave is the point on the medium that exhibits the maximum amount of negative or downward displacement from the rest position.
- The amplitude of a wave refers to the maximum amount of displacement of a particle on the medium from its rest position. In a sense, the amplitude is the distance from rest to crest. Similarly, the amplitude can be measured from the rest position to the trough position.
- The wavelength of a wave is simply the length of one complete wave cycle. If you were to trace your finger across the wave in the diagram above, you would notice that your finger repeats its path. A wave is a repeating pattern. It repeats itself in a periodic and regular fashion over both time and space. And the length of one such spatial repetition (known as a wave cycle) is the wavelength. The wavelength can be measured as the distance from crest to crest or from trough to trough. In fact, the wavelength of a wave can be measured as the distance from a point on a wave to the corresponding point on the next cycle of the wave.
- A longitudinal wave is a wave in which the particles of the medium are displaced in a direction parallel to the direction of energy transport. A longitudinal wave can be created in a slinky if the slinky is stretched out horizontally and the end coil is vibrated back-and-forth in a horizontal direction.
- A compression is a point on a medium through which a longitudinal wave is traveling that has the maximum density. A region where the coils are spread apart, thus maximizing the distance between coils, is known as a rarefaction.
- A rarefaction is a point on a medium through which a longitudinal wave is traveling that has the minimum density. Points A, C and E on the diagram above represent compressions and points B, D, and F represent rarefactions.
- The frequency, (f) of a wave refers to how often the particles of the medium vibrate when a wave passes through the medium. Given this definition, it is reasonable that the quantity frequency would have units of cycles/second, waves/second, vibrations/second, or something/second. Another unit for frequency is the Hertz (abbreviated Hz) where 1 Hz is equivalent to 1 cycle/second. If a coil of slinky makes 2 vibrational cycles in one second, then the frequency is 2 Hz.
- Period, (T) refers to the time that it takes to do something. When an event occurs repeatedly, then we say that the event is periodic and refer to the time for the event to repeat itself as the period. The period of a wave is the time for a particle on a medium to make one complete vibrational cycle. Period, being a time, is measured in units of time such as seconds, hours, days or years. The period of orbit for the Earth around the Sun is approximately 365 days; it takes 365 days for the Earth to complete a cycle.
- The speed of an object refers to how fast an object is moving and is usually expressed as the distance traveled per time of travel. In the case of a wave, the speed is the distance traveled by a given point on the wave (such as a crest) in a given interval of time.The SI unit of speed is m/s.
Wave equation
The wave equation shows the relationship between speed, wavelength and frequency of a wave.
The diagrams below show several "snapshots" of the production of a wave within a rope. The motion of the disturbance along the medium after every one-fourth of a period is depicted. Observe that in the time it takes from the first to the last snapshot, the hand has made one complete back-and-forth motion.
A period has elapsed. Observe that during this same amount of time, the leading edge of the disturbance has moved a distance equal to one complete wavelength. So in a time of one period, the wave has moved a distance of one wavelength. Combining this information with the equation for speed (speed = distance/time), it can be said that the speed of a wave is also the wavelength/period.
Since the period is the reciprocal of the frequency, the expression 1/f can be substituted into the above equation for period. Rearranging the equation yields a new equation of the form:
Speed = Wavelength • Frequency.The above equation is known as the wave equation. It states the mathematical relationship between the speed (v) of a wave and its wavelength (λ) and frequency (f). Using the symbols v, λ, and f, the equation can be rewritten asv = f • λ
Types of Waves
Identify types of waves
Previously we classified the waves by considering the movement of the particles. And now you’re going to find out the 2 types of waves according to the media of propagation.
- Mechanical Waves
- Electromagnetic Waves
Mechanical Waves
Mechanical waves are also called elastic waves as their propagation depends on the elastic properties of the medium through which the waves pass
Mechanical waves are divided into three categories: Transverse waves, longitudinal waves, and surface waves. In transverse waves, the medium moves perpendicular to the wave direction, and in longitudinal waves, the medium moves parallel to the wave direction.
In surface waves, both transverse and longitudinal waves mix in a single medium. In very simple words, an electronic wave is that which travels in a vacuum, and a mechanical wave is that which needs some medium for traveling.
Examples of mechanical waves are Sound waves, Water waves , Ocean waves, Earth quake waves, Seismic waves
Electromagnetic Waves
Electromagnetic waves are waves that have no medium to travel whereas mechanical waves need a medium for its transmission. Examples of electromagnetic waves include light and radio signals.
The following are the differences between mechanical and electromagnetic waves.
- Electromagnetic waves travel in a vacuum whereas mechanical waves do not.
- The mechanical waves need a medium like water, air, or anything for it to travel.
- While an electromagnetic wave is called just a disturbance, a mechanical wave is considered a periodic disturbance.
Behaviour of Waves
Reflection, Refraction, Diffraction and Interference of Waves
Explain Reflection, refraction, diffraction and interference of waves
All waves behave in certain characteristic ways. They can undergo:
- Reflection
- Refraction
- Diffraction
- Interference
These basic properties define the behaviour of a wave – anything that reflects, refracts, diffracts and interferes is labelled a wave.
Reflection
Reflection is the change in direction of a wavefront at an interface between two different media so that the wavefront returns into the medium from which it originated. Common examples include the reflection of light, sound and water waves.
The law of reflection
If the reflecting surface is very smooth, the reflection of light that occurs is called specular or regular reflection. The laws of reflection are as follows:
- The incident ray, the reflected ray and the normal to the reflection surface at the point of the incidence lie in the same plane.
- The angle which the incident ray makes with the normal is equal to the angle which the reflected ray makes to the same normal.
- The reflected ray and the incident ray are on the opposite sides of the normal.

Characteristics of Reflection of waves:
- It obeys the Law of Reflection.
- The wavelength, λ of the reflected wave is the same as that of the incident waves.
- The frequency,fof the reflected waves is the same as that of the incident waves.
- Therefore the speed, v of the reflected waves is the same as that of the incident waves.
Types of reflection:
- Specular: Smooth surfaces direct reflected light at opposite angle.
- Diffused: Rough surfaces scatter light in all directions.
- Spread: Some surfaces have a combination texture and smooth surface (varnish overcoat on paper, white label on white bottle).
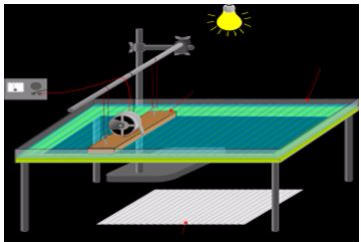
Ripple Tank
A ripple tank is a shallow glass tank of water used in schools and colleges to demonstrate the basic properties of waves. It is a specialized form of a wave tank. The ripple tank is usually illuminated from above, so that the light shines through the water.
Some small ripple tanks fit onto the top of an overhead projector, i.e. they are illuminated from below. The ripples on the water show up as shadows on the screen underneath the tank. All the basic properties of waves, including reflection, refraction, interference and diffraction, can be demonstrated.
Ripples may be generated by a piece of wood that is suspended above the tank on elastic bands so that it is just touching the surface. Screwed to wood is a motor that has an off centre weight attached to the axle. As the axle rotates the motor wobbles, shaking the wood and generating ripples.
Refraction
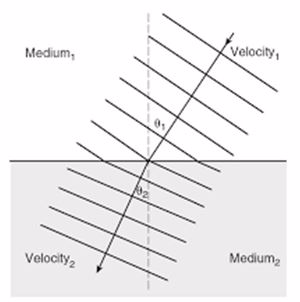
Refraction is the change in direction of propagation of a wave due to a change in its transmission medium.
The phenomenon is explained by the conservation of energy and conservation of momentum. Due to change of medium, the phase velocity of the wave is changed but its frequency remains constant. This is most commonly observed when a wave passes from one medium to another at any angle other than 0° from the normal.
Refraction of light is the most commonly observed phenomenon, but any type of wave can refract when it interacts with a medium, for example when sound waves pass from one medium into another or when water waves move into water of a different depth.
Snell’s law
Refraction is described by Snell's law, which states that: “For a given pair of media and a wave with a single frequency, the ratio of the sines of the angle of incidence θ1 and angle of refraction θ2 is equivalent to the ratio of phase velocities (v1 / v2) in the two media, or equivalently, to the opposite ratio of the indices of refraction (n2 / n1)”
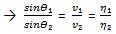
The refractive index or index of refraction n of an optical medium is a dimensionless number that describes how light, or any other radiation, propagates through that medium.
Refraction of a light ray.
The refractive index determines how much light is bent, or refracted, when entering a material. This is the historically first use of refractive indices and is described by Snell's law of refraction, n1 sin θ1 = n2 sin θ2, where θ1 and θ2 are the angles of incidence and refraction, respectively, of a ray crossing the interface between two media with refractive indices n1 and n2.
The refractive indices also determine the amount of light that is reflected when reaching the interface, as well as the critical angle for total internal reflection and Brewster's angle.
Interference
Interference is a phenomenon in which two waves superpose to form a resultant wave of greater or lower amplitude.
Interference usually refers to the interaction of waves that are correlated or coherent with each other, either because they come from the same source or because they have the same or nearly the same frequency.
Interference effects can be observed with all types of waves, for example, light, radio, acoustic, surface water waves or matter waves.
Constructive Interference
Constructive interference is a type of interference that occurs at any location along the medium where the two interfering waves have a displacement in the same direction.
In this case, both waves have an upward displacement; consequently, the medium has an upward displacement that is greater than the displacement of the two interfering pulses. Constructive interference is observed at any location where the two interfering waves are displaced upward. But it is also observed when both interfering waves are displaced downward.
This is shown in the diagram below for two downward displaced pulses.

-In this case, a sine pulse with a maximum displacement of -1 unit (negative means a downward displacement) interferes with a sine pulse with a maximum displacement of -1 unit. These two pulses are drawn in red and blue. The resulting shape of the medium is a sine pulse with a maximum displacement of -2 units.
Destructive Interference
Destructive interference is a type of interference that occurs at any location along the medium where the two interfering waves have a displacement in the opposite direction.
For instance, when a sine pulse with a maximum displacement of +1 unit meets a sine pulse with a maximum displacement of -1 unit, destructive interference occurs.
Diffraction
Diffraction refers to a change in direction of waves as they pass through an opening or around a barrier in their path.
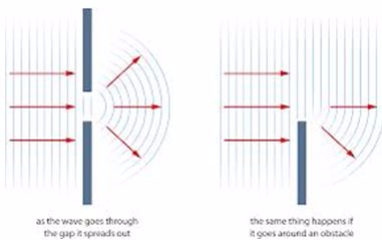
In classical physics, the diffraction phenomenon is described as the interference of waves according to the Huygens–Fresnel principle. These characteristic behaviors are exhibited when a wave encounters an obstacle or a slit that is comparable in size to its wavelength. Similar effects occur when a light wave travels through a medium with a varying refractive index, or when a sound wave travels through a medium with varying acoustic impedance.
Diffraction occurs with all waves, including sound waves, water waves, and electromagnetic waves such as visible light, X-rays and radio waves. Diffraction arises because of the way in which waves propagate; this is described by the Huygens–Fresnel principle and the principle of superposition of waves.
The propagation of a wave can be visualized by considering every particle of the transmitted medium on a wavefront as a point source for a secondary spherical wave. The wave displacement at any subsequent point is the sum of these secondary waves.
When waves are put together, their sum is determined by the relative phases as well as the amplitudes of the individual waves so that the summed amplitude of the waves can have any value between zero and the sum of the individual amplitudes.
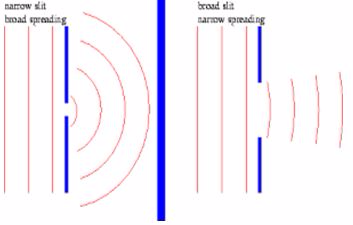
A long slit of infinitesimal width which is illuminated by light diffracts the light into a series of circular waves and the wavefront which emerges from the slit is a cylindrical wave of uniform intensity.A slit which is wider than a wavelength produces interference effects in the space downstream of the slit. These can be explained by assuming that the slit behaves as though it has a large number of point sources spaced evenly across the width of the slit
Diffraction of water waves is observed in a harbor as waves bend around small boats and are found to disturb the water behind them. Diffraction of sound waves is commonly observed; we notice sound diffracting around corners, allowing us to hear others who are speaking to us from adjacent rooms.
Diffraction is observed of light waves but only when the waves encounter obstacles with extremely small wavelengths (such as particles suspended in our atmosphere).
The Application of Reflection, Refraction, Diffraction and Interference of Waves in Daily Life
Mention the application of reflection, refraction, diffraction and interference of waves in daily life
Application of reflection of waves
- The phenomenon of the reflection of sound is used to determine the distance between the two objects, for example depth of seabed, depth of cave or width of a valley. The type of sound used must be ultrasound.
- Sonar (Sound Navigation and Ranging). Sonar is used to detect underwater objects (corals / fishes) or to determine the depth of the water by means of an echo. Sonar equipment emits a high frequency sound signal which is reflected by the object in the water. The reflected sound wave is received by the sonar receiver. The time taken for the echo to return is used to determine the distance of the object below the water surface. Sonar waves of high frequency is used because itpossessesmore energy, high penetration power and can travel further through water.
- Reflection of light waves is used in the design of mirrors.
- Detection of cracks in metals.
- Determination of frequency of A.C’s.
Applications of Refraction
- Refraction has many applications in optics and technology. A lens uses refraction to form an image of an object for many different purposes, such as magnification.
- A prism uses refraction to form a spectrum of colors from an incident beam of light.
- Refraction also plays an important role in the formation of a mirage and other optical illusions.
Applications of interference of waves
- Interference is applied when creating holograms. A hologram is a photograph of an interference pattern which is able to produce a three-dimensional image when suitably illuminated.
- Destructive interference is used in noise reduction systems such as earphones.The system capture sound from the environment and use computer technology to produce a second sound wave,which leads to reduction in the loudness of the noise.
- Concert halls and auditorium are usually designed in such a way to reduce the amount of destructive interference. Usually, the walls and ceiling made in such a way that they absorb rather than reflect sound.
Application of wave diffraction
- Diffraction Grating: A diffraction grating is an optical device that consists of not one but many thousands of apertures. Spectra produced by diffraction gratings are extremely useful in applications from studying the structure of atoms and molecules to investigating the composition of stars.
- X-ray diffraction: X rays are light waves that have very short wavelengths. When they irradiate a solid, crystal material they are diffracted by the atoms in the crystal.x-ray diffraction utilises an instrument called a diffractometer to produce diffraction patterns that can be compared with those of known crystals to determine the structure of new materials.
- Holography: Holography is the science and practice of making holograms. Normally, a hologram is a photographic recording of a light field, rather than of an image formed by a lens, and it is used to display a fully three-dimensional image of the holographed subject, which is seen without the aid of special glasses or other intermediate optics. An illuminating laser beam is diffracted at specific angles, in accordance with Bragg's law, on the surfaces of the hologram, making it possible for an observer to see a three-dimensional image.
The Behaviour of Waves
Demonstrate the behaviour of waves
Activity 1
Demonstrate the behaviour of waves
Behaviour of Waves are reflection, refraction, diffraction and interference of Waves. All waves behave in certain characteristic ways. They can undergo: Reflection, Refraction, Diffraction and Interference.These basic properties define the behaviour of a wave – anything that reflects, refracts, diffracts and interferes is labeled as a wave.
Propagation of Waves
The Propagation of Mechanical Waves
Describe the propagation of mechanical waves
Mechanical waves can be divided into three main categories according to the ways in which they travel, known aspropagation. The three propagation types are:
- Atransverse waveis one that vibrates at 90 degrees to the direction the wave is moving. For example, if you hold a Slinky between two hands and shake it up and down, you'll get a wave that moves along the Slinky, but the vibrations will still be up and down. Underwater waves are also transverse.

Transverse wave
- Alongitudinal waveis one in which the vibrations are parallel to the direction the wave is moving. That's like sending a pulse along the length of a Slinky, pushing it lengthwise. Instead of peaks and troughs, longitudinal waves havecompressions(areas where the Slinky is bunched together), andrarefactions(areas where the Slinky is spread apart). Another example of a longitudinal wave is a sound wave. Although you can't see air molecules, if you could, you would notice that sound involves air molecules hitting each other, thereby producing areas with high densities of molecules (compressions) and areas with low densities of molecules (rarefactions).
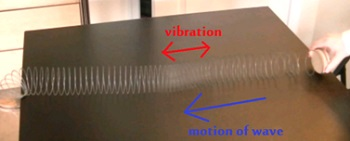
Longitudinal wave
- Last of all, asurface waveis a wave that travels along the boundary between two materials. For example the kind of water wave you most often see--along the top of water--is an example of a surface wave. Surface waves move in similar ways to transverse waves but are a bit more complicated in their behavior.

Surface wave
In the case of an earthquake, you get a mixture of all three types of waves. The initial earthquake (called the primary wave) is longitudinal, but the aftershock that comes later (called the secondary wave) is transverse. Extra surface waves are also created.
The Propagation of Electromagnetic Waves
Explain the propagation of electromagnetic waves
Electromagnetic waves are transverse waves only. Velocity of all electromagnetic waves is equal to the velocity of light which is 300,000,000 m/s (3x10exp8). Electromagnetic waves do not need medium for their transmission. Electromagnetic waves are arranged in special arrangement known as electromagnetic spectrum according to the increase in their frequencies or decrease in their wavelengths.
The Relationship between Frequency, Speed and Wavelength of a Wave
Determine the relationship between frequency, speed and wavelength of a wave
Frequency (f) of a wave is inversely proportional to its wavelength (l). Speed (V) of a wave is constant. That is, f=V/l. That is Speed of a wave can be expressed as a product of frequency and wavelength. That is, V = f X l Speed of a wave is expressed in meters per second (m/s). Period (T) of a wave is a time taken by a wave to complete one oscillation. Frequency is a reciprocal of the Period.
Mathematically it can be shown as:v = f • λ
where
- v =speed of a wave
- λ =wavelength
- f = frequency
The Refractive Index of a Medium
Determine the refractive index of a medium
Refractive index(n) is a ratio of the velocity (Va) of a wave in air to its velocity in a medium (Vm). n=Va/Vm. Refractive index can also be obtained as a ratio of the sine of angle of incidence (Sin i) to the sine of angle of refraction (Sin r). The speed of the wave depends on the medium through which it travels. Changing the medium changes the speed. Absolute refractive index=V in vacuum/V.
Refractive index of a material is a measure of the change in speed of light as it passes from a vacuum (or air as an approximation) into the material.

In the equation above,v1is the speed of light in a vacuum. The bigger the refractive index the slower the light travels in that material - i.e. the smallerv2is.
Example 1
Light of frequency 4.6 × 1014Hz travels at a speed of 1.24 × 108ms-1in diamond.Calculate the refractive index of diamond for this colour of light.
Solution
vdiamond= 1.24 × 108ms-1
c= 3.0 × 108ms-1
(from data sheet)


Refractive index of diamond for this colour of light = 2.42
Sound Waves
Source of Sound Wave
Identify source of sound waves
Sound is oscillation in pressure, stress, particle displacement, particle velocity, etc., propagated in a medium with internal forces (e.g., elastic or viscous), or the superposition of such propagated oscillation.
The sources of sound
- vibrating solids.
- rapid expansion or compression (explosions and implosions).
- Smooth (laminar) air flow around blunt obstacles may result in the formation of vortices (the plural of vortex) that snap off or shed with a characteristic frequency. This process is called vortex shedding and is another means by which sound waves are formed. This is how a whistle or flute produces sound. Also the aeolian harp effect of singing power lines and fluttering venetian blinds.
The Concept of Audibility
Explain the concept of audibility range
Audibility range the range of frequencies that can be heard by humans or other animals, though it can also refer to the range of levels.
The human range is commonly given as 20 to 20,000Hz, though there is considerable variation between individuals, especially at high frequencies, and a gradual loss of sensitivity to higher frequencies with age is considered normal
Sensitivity also varies with frequency, as shown by equal-loudness contours. Routine investigation for hearing loss usually involves an audiogram which shows threshold levels relative to a normal. Several animal species are able to hear frequencies well beyond the human hearing range. Some dolphins and bats, for example, can hear frequencies up to 100kHz.
Several animal species are able to hear frequencies well beyond the human hearing range. Some dolphins and bats, for example, can hear frequencies up to 100kHz.
The Perception of Hearing
Describe the perception of hearing
A basic measure of hearing is afforded by an audiogram: a graph of the minimum discernible sound level at various frequencies throughout an organism's nominal hearing range.
Behavioural hearing tests or physiological tests can be used to find hearing thresholds of humans and other animals. For humans, the test involves tones being presented at a specific frequencies (pitch) and intensities (loudness). When the subject hears the sound, he or she indicates it by raising a hand or pressing a button. The lowest intensity they can hear is recorded.
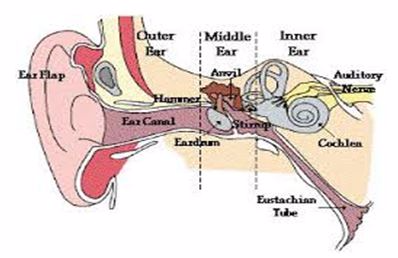
The human ear
The ear is the organ that detects sound. It not only receives sound, but also aids in balance and body position. The ear is part of the auditory system.
Often the entire organ is considered the ear, though it may also be considered just the visible portion. In most mammals, the visible ear is a flap of tissue that is also called the pinna (or auricle in humans) and is the first of many steps in hearing.
Vertebrates have a pair of ears placed somewhat symmetrically on opposite sides of the head. This arrangement aids in the ability to localize sound sources.
The human ear is divided into three parts:
The outer ear
The outer ear comprises the pinna (auricle), which is made of a convoluted plate of flexible cartilage that extends as a nearly closed tube one-third of the way down the ear canal. This outer third, which is about eight millimetres (one-third of an inch) long, has small hairs that point outwards to form a line of defence against small animals creeping in. The roots of the hairs produce oils and these mix with the secretions from nearby sweat-like glands to form the basis of wax.
The deep two-thirds of the ear canal (16 millimetres/two-thirds of an inch long) has a bony wall lined with thin and rather fragile skin which is devoid of glands. At the far end of the ear canal and stretched across it is the eardrum (tympanic membrane), which forms the boundary between the outer and middle ears.
The middle ear (Tympanum)
The eardrum is a circle of thin skin about eight to nine millimetres (one-third of an inch) in diameter. Despite its name, it is not flat like the skin of a drum, but is slightly conical with the curved sides sloping inwards. The eardrum has three layers.
The inner ear (Labyrinth)
The inner ear is probably the most remarkably intricate piece of the body. It makes hearing possible by converting sound into electrical impulses that then travel along the hearing nerve (the acoustic nerve or auditory nerve) to the brain. The inner ear also plays a major role in balance. The balance portions of the inner ear (vestibular labyrinth) can detect acceleration of the head in any direction whether in a straight line (linear) or twisting and turning (angular). The electrical signals that arise in response to head movement pass along the balance nerve (vestibular nerve), which in due course joins with the hearing nerve to form a single bundle (statoacoustic, vestibulo-acoustic or eighth nerve, nerve VIII) which then enters the brain.
The portion of the inner ear that actually hears is the cochlea. This is a hollow coiled tube set in the very dense bone called the bony labyrinth (part of the petrous [rocklike] temporal bone). This tube is filled with fluid, which is much the same as general body fluid (lymph) and that which surrounds the brain (cerebrospinal fluid – CSF). This inner-ear fluid is called perilymph. Inside the perilymph is another coiled triangular-shaped tube called the cochlear duct (scala media), which contains the all-important ‘hair cells’ – these convert sound into electricity. These hair cells are arranged in two groups that follow the coils of the cochlear duct and spiral upwards from base to apex. There is a single row of inner hair cells (IHCs), which lie closer to the core of the cochlea (modiolus), and three or four rows of outer hair cells (OHCs), which are further away. In a healthy young human ear there are about 3,500 IHCs and about 12,000 OHCs. Each hair cell has a cluster of small rigid hairs (stereocilia), which project from the thicker upper surface of the cell into the special fluid that fills the cochlear duct. This fluid is called endolymph and is remarkable in that it has a strongly positive electrical charge associated with it – about 80 millivolts – and is rich in potassium, a metallic element.
The hair cells in their rows are grouped together with their supporting cells in the organ of Corti. This is a small ridge that sits on a thin, very flexible membrane called the basilar membrane. The basilar membrane forms the floor of the triangular cochlear duct. The sloping roof is another very thin membrane (Reissner’s membrane) and the side wall is a thickened region rich in blood vessels (the stria vascularis). This structure is responsible for maintaining the composition of the rather unusual and very important endolymph.
Adjacent to the base of the hair cells are the nerves that carry impulses to the brain (the afferent nerves). At least 90 per cent of these nerves come from the inner hair cells, despite their smaller number. Each inner hair cell has about 10 nerve endings attached to it and there are, therefore, about 30,000 nerve fibres in the acoustic nerve.
The hearing nerves travel inwards, along with the balance and facial nerves, through a canal in the inner part of the skull (variously called the internal auditory meatus [IAM], internal auditory canal [IAC] or porus acousticus) to reach the brain stem. This part of the brain deals with lots of automatic functions such as pulse, blood pressure, general alertness, balance, and so on.
About half of the hearing nerves from each ear cross over to the other side of the brain stem and then, on both sides, the nerves pass up the brain stem through the midbrain, eventually to reach ‘consciousness’ in what is called the cortex of the brain. For hearing, this conscious region is located in the temporal lobe portion of the brain, which lies on each side of the head just above the ear.
The Concept of Echo and Reverberation
Explain the concept of echo and reverberation
Echo
An echo occurs when asound wave is reflected and hence arrives to the listener after some time delay after the direct sound.
When we are in a empty space surrounded by mountains and we shout loudly, we see that faint sound resembling the original sound coming back after some delay of time. This perception of the reflected wave is nothing but the echo. This is experienced in remote places, in rooms that are big and empty, in caves, buildings
Echo is derived from the Greek word which means Sound.The echo is produced due to hitting of the sound waves with the obstacles which makes the sound to reflect back

Application of Echo:
Measuring distance
By knowing the speed of sound and measuring the time it takes to hear the echo, you can calculate the distance of the object. A sonar device sends out a sound and automatically calculates the distance of an object. Submarines use sonar to find objects under the water, including other submarines. The "ping" sound heard in a submarine comes from the sonar device sending out a sound wave under water.
Fishermen also use sonar to find schools of fish. Since this is an electronic device, the time it takes for the wave to return can be much less than the 0.1 second required to hear an echo. For example, if the speed of sound in water is 1500 m/s and the fisherman's sonar device detects an echo in 0.02s, the distance of the object under water will bed = v x t = 1500 m/s x 0.02s = 30 m (back and forth).
That may mean a school of fish are 15 meters away. Sonar and radar work on the same principle. Sonar uses sound waves, while radar uses electromagnetic waves.
Velocity
When a wave bounces off a moving object, the frequency of the sound changes, according to the relative velocity of the object. (Velocity is the measurement of speed and direction). If the object is moving toward you, the frequency or pitch of the sound gets higher. When it is moving away, the pitch gets lower. The faster the object is moving, the greater the change in frequency or pitch. This is called the Doppler Effect.
You have probably experienced the Doppler Effect when you heard how the sound of an ambulance siren changes pitch as it passes by. The Doppler Effect can be used to measure the velocity of an object by comparing the frequency of the sound sent out to the frequency of the sound reflected by in the echo. A sonar device is usually used to calculate the velocity of the object.
Note: Doppler radar works on a similar principle to measure the speed of storms in weather prediction, except that it uses echoes from electromagnetic waves.
Bats can find moths
Bats use echoes to find good tasting moths, while flying around at night. The bat sends a sharp click or chirping sound and then hears and processes any echoes off other objects in the area. Bats have large ears that are very sensitive to sounds in certain wavelengths
Reverberation
Reverberations are the collection of reflected sounds from the surfaces in an enclosure like an auditorium. It is a desirable property of auditoriums to the extent that it helps to overcome the inverse square law drop off of sound intensity in the enclosure.
However, if it is excessive, it makes the sounds run together with loss of articulation - the sound becomes muddy, garbled.

A reverberation is the same as echo but the distance here is less.The distance between the source of the sound and the obstacle by which it is reflected is less in Reverberation.Here, in reverberation the time delay is less than 0.1 second.
The reflected wave reaches the observer in less than 0.1 second. Now as the delay in time is less than the original sound is still in memory, the delay between perception of sound and the original sound is very-very less.
Question Time 1
How is an Echo Different from the reverberation?
Echo is when long distances are considered and reverberation is when short distances are considered. Echo is due to the reflection of sound wave by obstacles or end points like wall etc. But Reverberation is due to the collection of reflection sounds from the surface which is enclosed completely.
The Speed of Sound in Air
Determine the speed of sound in air
Sound can travel in air (gases), liquids and in solids. Sound is a mechanical wave hence can not travel in vacuum. The speed of sound in air is approximately 340 m/s. The speed of sound in air changes with temperature. As the temperature increase the speed of sound also increases. Sound travel faster in solids than in liquids and air.
Musical Sound
The Concept of a Musical Sound
Explain the concept of a musical sound
Music is organised sound which has some pattern. Music uses certain frequencies or combinations of frequencies called the musical scale to produce sounds that are generally appealing to the human ear.
Noise on the other hand, is random and without structure. Any and all frequencies might occur and their combination is often not appealing to the ear.
Factors Affecting Loudness, Pitch and Quality of Musical Sound
Identify factors affecting loudness, pitch and quality of a musical sound
The musical sounds produced by different musical instruments have distinct properties that are used to describe them. These include loudness, pitch and timbre:
- Loudness:loudness is the intensity of the sound which is the perceptual property. It is determined by the amplitude of sound wave and the number of auditory nerves activated by sound wave. Amplitude is a physical property determined by how much air pressure in a compression or rarefaction deviates from normal air pressure. The larger the amplitude the louder the sound.
- Pitch is an auditory sensation in which a listener assigns musical tones to relative positions on a musical scale based on the frequency of sound wave vibration. Frequency is an objective, scientific concept, whereas pitch is subjective. Sound waves themselves do not have pitch. It takes a human brain to map the internal quality of pitch.-Pitches are usually quantified as frequencies in cycles per second, or hertz.
- Timbre is the tone quality of sound produced by an instrument. It is referred to as sound quality or sound colour and it is a perceptual property. What makes a particular musical sound different from another, even when they have the same pitch and loudness.
Different Musical Instruments
Identify the different musical instruments
Musical instrument are the device constructed or modified for the purpose of making music. They are categorizied into three categories:
- Wind Instruments:This class of musical instruments requires you to blow into a specific wind instrument by following an order to ensure that the sound that you desire is produced. The instruments can be expected to work depending on the principles of frequencies, sound waves, acoustics, resonance and harmonics. The pitch of the produced sound when you start blowing the instrument is actually dependent on the length of the air column through which the waves of the sounds vibrate.Some of the most popular wind instruments are piccolo, flute, clarinet, shakuhachi, bassoon, oboe, accordion, English horn, harmonica, saxophone, pianica, bagpie and shehnai.
- Percussion Instruments:These instruments require you to strike the surface of the instrument to generate vibrations to produce your desired note. Percussion instruments can actually be divided into two types. The first type includes tuned instruments that are known to produce a definite pitch or a series of different pitches. Some examples of the tuned percussion instruments include xylophone, vibraphone, marimba, tubular bells and timpani or kettle drum. The second type of percussion instruments is the indefinite pitch. Its examples include triangle, castanets, rattle, cymbals, tambourine, anvil and gong.
- String Instruments: These are composed of those instruments that work based on sound wave vibrations produced by strings. The pitch that can be produced by these instruments is dependent on the length of air column and the type and thickness of strings used.Among the most popular string instruments are guitar, viola, violin, cello, mandolin, harp, double bass and banjo.
The Terms Stationary Wave, Nodes and Antinodes
Explain the terms stationary wave, nodes and antinodes
Stationary wave
Is a wave in a medium in which each point on the axis of the wave has an associated constant amplitude
This phenomenon can occur because the medium is moving in the opposite direction to the wave, or it can arise in a stationary medium as a result of interference between two waves traveling in opposite directions.

The most common cause of standing waves is the phenomenon of resonance, in which standing waves occur inside a resonator due to interference between waves reflected back and forth at the resonator's resonant frequency.
For waves of equal amplitude traveling in opposing directions, there is on averageno net propagation of energy.Traveling waves have high points called crests and low points called troughs (in the transverse case) or compressed points called compressions and stretched points called rarefactions (in the longitudinal case) that travel through the medium.
Standing waves don't go anywhere, but they do have regions where the disturbance of the wave is quite small, almost zero. These locations are called nodes. There are also regions where the disturbance is quite intense, greater than anywhere else in the medium, called antinodes.
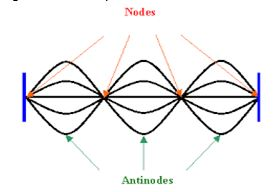
Nodes
The locations at which the amplitude is minimum are called nodes.
Antinodes
The locations where the amplitude is maximum are called antinodes.
The Frequency of a Musical Note
Determine the frequency of a musical note
The frequency of a musical note is affected by length of vibrating string and the velocity of the waves. Velocity of the waves depends on the tension on the string and the linear mass density. The linear mass density is the mass per unit length. The frequency of a music note depends on the length, mass per unit length and the tension.
The Difference between the Fundamental Note and Overtones
Distinguish between the fundamental note and overtones
Fundamental Note
Fundamental note is the lowest resonant frequency of a vibrating object. Most vibrating objects have more than one resonant frequency and those used in musical instruments typically vibrate at harmonics of the fundamental.
A harmonic is defined as an integer (whole number),n multiple of the fundamental frequency. Vibrating strings, open cylindrical air columns, and conical air columns will vibrate at all harmonics of the fundamental.
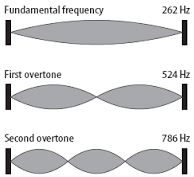
Overnote
An overtone is any frequency higher than the fundamental frequency of a sound. Using the model of Fourier analysis, the fundamental and the overtones together are called partials.Harmonics, or more precisely, harmonic partials, are partials whose frequencies are integer multiples of the fundamental (including the fundamental which is 1 times itself). These overlapping terms are variously used when discussing the acoustic behavior of musical instruments.
There are integer multiples of a certain frequency (fundamental), that are called harmonics, partial tones (partials) or overtones.It is important to note that the term 'overtones' does not include the fundamental frequency. The first overtone is therefore already the second harmonic or the second partial. The term overtone should never be mixed with the other terms, as the counting is unequal.The term harmonic has a precise meaning - that of an integer (whole number) multiple of the fundamental frequency of a vibrating object.
The Concept of Resonance as Applied to Sound
Explain the concept of resonance as applied to sound
Resonance is a phenomenon that occurs when a given system is driven by another vibrating system or external force to oscillate with greater amplitude at a specific preferential frequency.Frequencies at which the response amplitude is a relative maximum are known as the system's resonant frequencies, or resonance frequencies.
At resonant frequencies, small periodic driving forces have the ability to produce large amplitude oscillations. This is because the system stores vibrational energy. Resonance occurs when a system is able to store and easily transfer energy between two or more different storage modes (such as kinetic energy and potential energy in the case of a pendulum).
However, there are some losses from cycle to cycle, called damping. When damping is small, the resonant frequency is approximately equal to the natural frequency of the system, which is a frequency of unforced vibrations. Some systems have multiple, distinct, resonant frequencies.
Resonance in Closed Ended Pipes
A closed ended instrument has one end closed off, and the other end open. An example would be an instrument like some organ pipes (although in some designs they are open), or a flute.
Although you blow in through the mouth piece of a flute, the opening you’re blowing into isn’t at the end of the pipe, it’s along the side of the flute. The end of the pipe is closed off near the mouth piece. Remember that it is actually air that is doing the vibrating as a wave here.
- The air at the closed end of the pipe must be a node (not moving), since the air is not free to move there and must be able to be reflected back.
- There must also be an antinode where the opening is, since that is where there is maximum movement of the air.
- The simplest, smallest wave that I can possibly fit in a closed end pipe is shown in Figure below.
- Notice how even though it has been flipped left-to-right and it looks squished and stretched a bit to fit, this is still ¼ of a wavelength.
- Since this is the smallest stable piece of a wave I can fit in this pipe, this is the Fundamental, or 1st Harmonic.
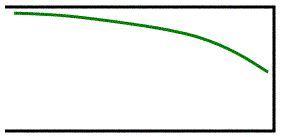
Since the length of the tube is the same as the length of the ¼ wavelength I know that the length of this tube is ¼ of a wavelength… this leads to our first formula: L = ¼ λ
- “L” is the length of the tube in meters. On it’s own this formula really doesn’t help us much.
- Instead, we have to solve this formula for λ and then combine it with the formula v=fλ to get a more useful formula:
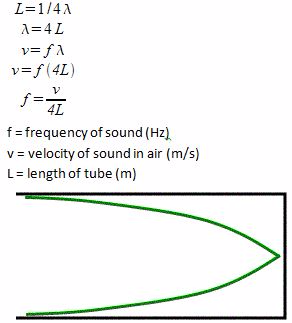
When the wave reaches the closed end it’s going to be reflected as an inverted wave (going from air to whatever the pipe is made of is a pretty big change so this is what we would expect). It would look like Figure above.
This does not change the length of the wave in our formula, since we are only seeing the reflection of the wave that already exists in the pipe.
What does the next harmonic look like? It’s the 3rd Harmonic.
- I know this name might seem a little confusing (I’m the first to agree with you!) but because of the actual notes produced and the way the waves fit in, musicians refer to the next step up in a closed end pipe instrument as the 3rd harmonic… there is no such thing as a 2nd harmonic for closed end pipes.
- In fact, all of the harmonics in closed end pipes are going to be odd numbers.
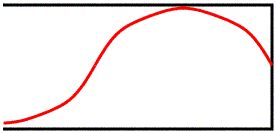
Remember that we have to have an antinode at the opening (where the air is moving) and a node at the closed end (where the air can’t move). That means for the 3rd harmonic we get something like Figure above. This is ¾ of a wavelength fit into the tube, so the length of the tube is… L = ¾ λ
This is the third harmonic of the closed end pipe. The formula for the frequency of the note we will hear is…
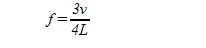
Do you notice a pattern forming in the formulas? Hopefully, because for both open and closed end pipes, we will only give you the formulas for the fundamentals lengths. You need to remember how to get the rest.
If we drew in the reflection of the third harmonic it would look like Figure below.
One more to make sure you see the pattern. The 5th Harmonic (fig. above)
There is one full wavelength in there (4/4) plus an extra ¼ of a wavelength for a total of 5/4. The length of the pipe is… L = 5/4 λ And the note produced by the 5th Harmonic is found using the formula…f = 5v/4L
Figure below shows the reflection of a 5th Harmonic for a closed end pipe.
Open End Pipes
An open ended instrument has both ends open to the air. An example would be an instrument like a trumpet. You blow in through one end and the sound comes out the other end of the pipe.

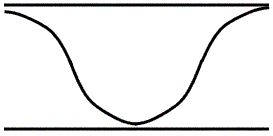
Fundamental
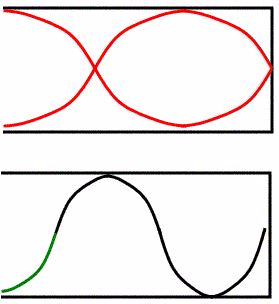
- The fundamental (first harmonic) for an open end pipe needs to be an antinode at both ends, since the air can move at both ends.
- That’s why the smallest wave we can fit in is shown in Figure 11.
- This looks different than the ½ wavelength that I showed you in Figure 3, but it is still half of a full wavelength.
- That means the length of the tube and frequency formulas are…L = ½ λ
f = v/2L
2nd Harmonic
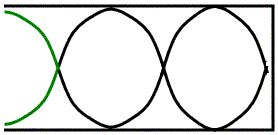
The next note we can play is the 2nd harmonic.
- Yep, open end pipes have a 2nd harmonic… they can have any number harmonic they want, odd or even.
- Again, it kind of looks weird, but trace it out and you’ll see that there is exactly one wavelength here.
- The length and frequency formulas are…L = 2/2 λ, f= 2v/2L
I’m not going to show you what the 3rd harmonic looks like. Instead, try drawing it yourself and see what you get.
As a hint to help you, the formulas for the length and frequency are…L = 3/2 λ, f = 3v/2L
A Simple Musical Instrument
Construct a simple musical instrument
A musical instrument is a device constructed for making music. There are three categories of musical instruments: string, percussion and wind instruments. The note of a musical instrument depends on five parameters: pitch, frequency, intensity, loudness and quality of a music note. Pitch of a note is its position in the musical scale and depends on the frequency of the vibration of the wave.
Activity 2
Construct a simple musical instrument
Electro-magnetic Spectrum
The Concept of Electro-magnetic Spectrum
Explain the concept of the electro-magnetic spectrum
The electromagnetic spectrum is the range of all possible frequencies of electromagnetic radiation.The "electromagnetic spectrum" of an object has a different meaning, and is instead the characteristic distribution of electromagnetic radiation emitted or absorbed by that particular object
The electromagnetic spectrum extends from below the low frequencies used for modern radio communication to gamma radiation at the short-wavelength (high-frequency) end, thereby covering wavelengths from thousands of kilometers down to a fraction of the size of an atom. The limit for long wavelengths is the size of the universe itself, while it is thought that the short wavelength limit is in the vicinity of the Planck length.
Properties of electromagnetic spectrum
- It is continuous i.e each band merges into the next and there are no gaps in the frequencies. The different kinds of radiation gradually change from one to another as their properties also gradually change.
- In some case, there is an overlap in the range of wavelength. This is because sometimes the name given to the wave(radiation) is determined by the source and not the wavelength(or frequency) for example x-rays and γ -rays.
The Main Bands of the Electro Magnetic Spectrum
Identify the main bands of the electroMagnetic spectrum
Radio waves
Radio waves are a type of electromagnetic radiation with the longest wavelengths in the electromagnetic spectrum. Radio waves have frequencies from 300GHz to as low as 3kHz, and corresponding wavelengths ranging from 1 millimeter (0.039in) to 100 kilometers (62mi).
Like all other electromagnetic waves, they travel at the speed of light. Naturally occurring radio waves are made by lightning, or by astronomical objects. Artificially generated radio waves are used for fixed and mobile radio communication, broadcasting, radar and other navigation systems, communications satellites, computer networks and innumerable other applications.
Radio waves are generated by radio transmitters and received by radio receivers.
Sources of radio waves
- alternating electric currents flowing in antennae
- oscillators
- planets, comets, stars and galaxies
Microwaves
Microwaves have a short wavelength of between 10⁻⁴m to about 0.1.
Sources
- Are produced by oscillation of charges in special antennae mounted on dishes.
- Magnetrons.
Infrared waves.
They have the frequency 0f between 10 and 10 Hz. They lie between the visible light and microwaves. They have the heating effect.
Sources: Vibration of atoms and molecules due to their thermal energy. All hot bodies emit infrared radiations.
Visible light.
Is the narrow range of EW frequencies to which human eyes are sensitive.
Detection of Infra-red, Visible and Ultra-violet Rays
Detect infra-red, visible and ultra-violet rays
Infrared waves
Infrared radiation is invisible to the human eye. However any nocturnal animal can “see” infrared radiations. We can sense infrared radiations as heat. Devices used to detect infrared radiations include black bulb thermometers, photographic films, thermistors and phototransistors.
Visible light
We see things because they either emit visible light or because they reflect visible light from another source.
Ultraviolet light.
Ultraviolet is light is detected by Photographic films; Fluorescent materials
Application of Electro-magnetic Wave in daily life
The Application of Micro-Waves, Radio-Waves, Gamma Rays and X-rays
Identify the application of micro-Waves, radio-Waves, Infra-red, gamma rays and x-rays
Radio waves
- Fixed and mobile radio communication, broadcasting, radar and other navigation systems, communications satellites, computer networks and innumerable other applications.
- Astronomers use large radio telescopes to collect and study radio waves from distant stars and galaxies. This helps them to determine composition, structure and motion of the celestial bodies.
Microwaves
- In cooking
- Radar systems
- Long distance communication
Gamma rays
- Medical application the same as x-rays
- In agriculture
X-rays
- X-ray photography
- Diagnosis and treatment of cancer
The Importance of Electro-magnetic Waves in Agriculture and Climate
Explain the importance of electro-magnetic waves in agriculture and climate
Electromagnetic radiations are very useful in agriculture and climate as they support photosynthesis, the main source of oxygen in the atmosphere. Gamma rays are used for pest control by sterilizing male pests with gamma radiation then releasing them into the wild, as is done to control tsetse flies. Gamma rays are used to obtain disease-resistant plants. Ultraviolet can purify water and air.
- READ TOPIC 2: Electromagnetism



No comments:
Post a Comment